XXXX秋季第3讲机械制图北京航空航天大学出版社

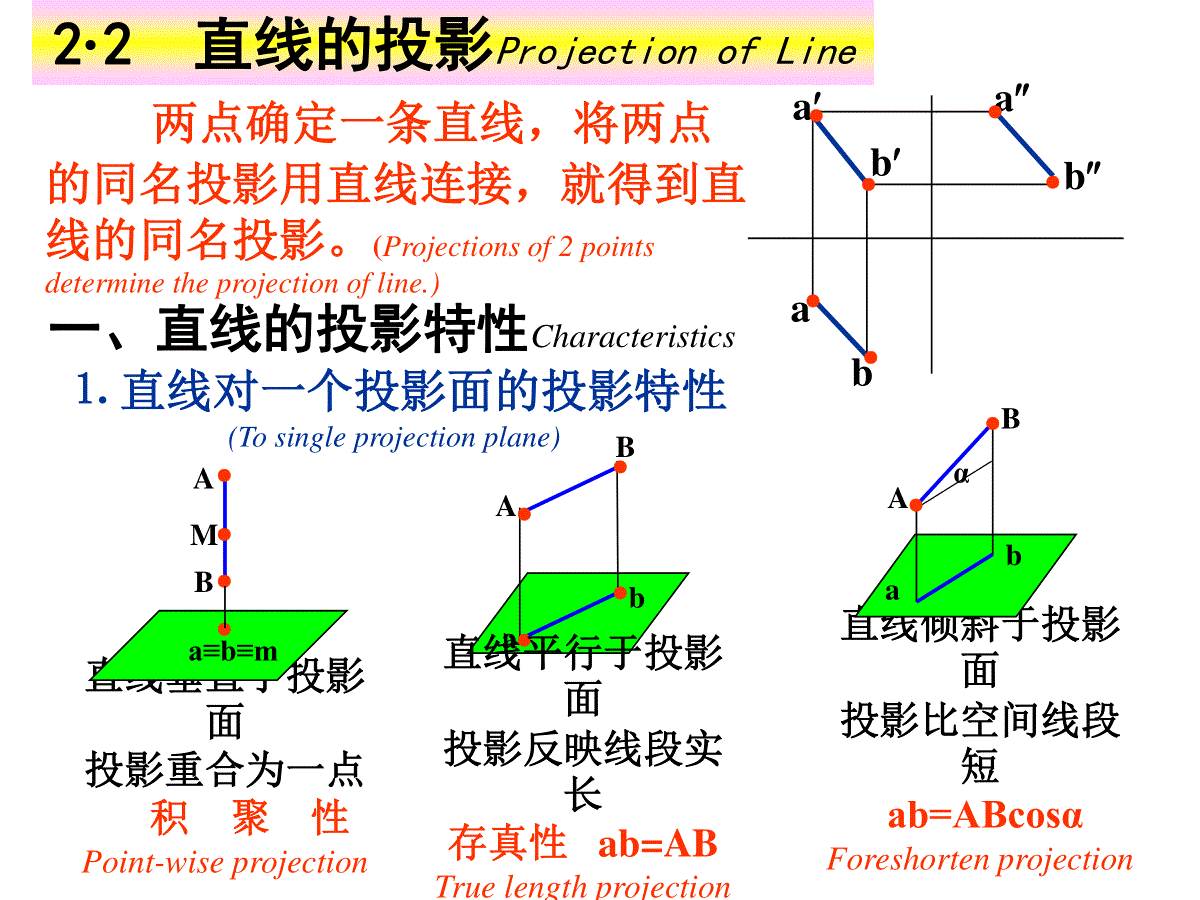
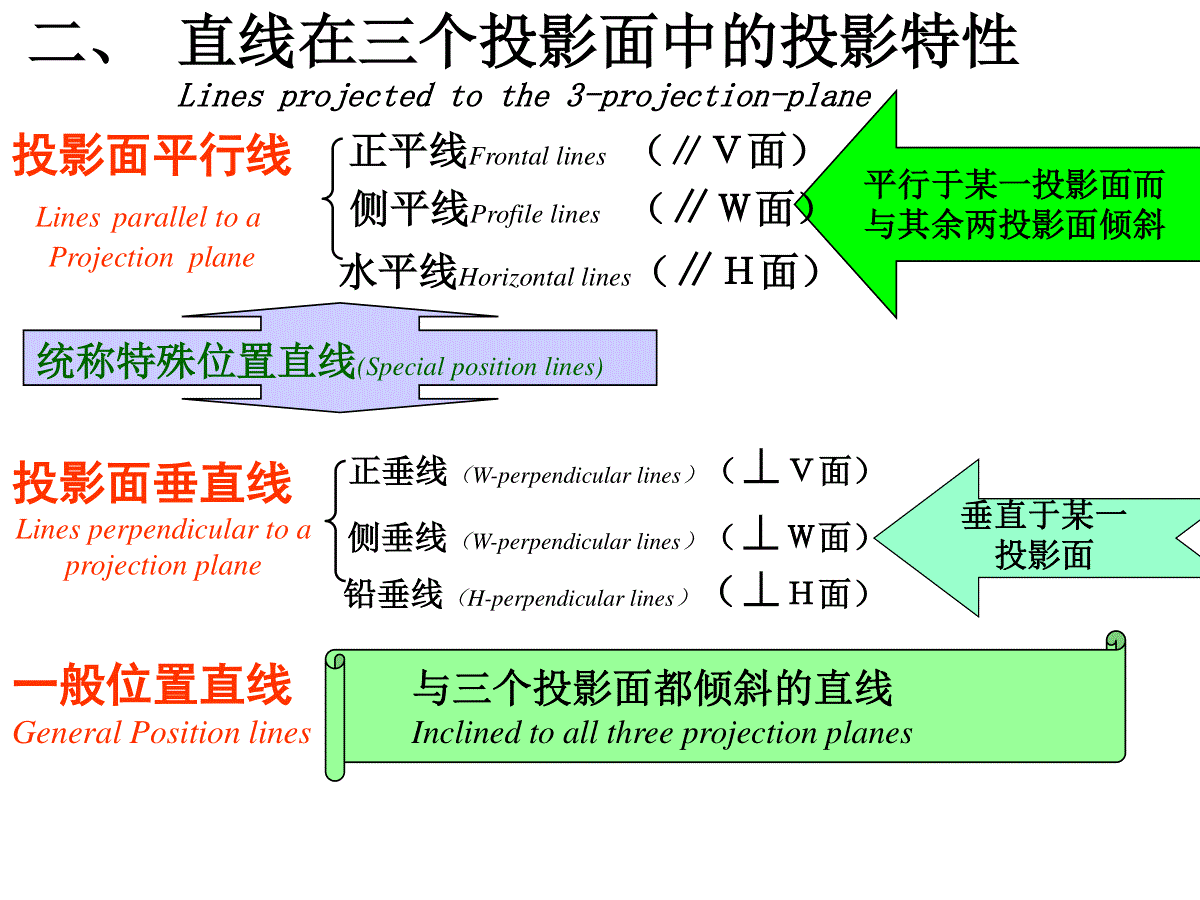
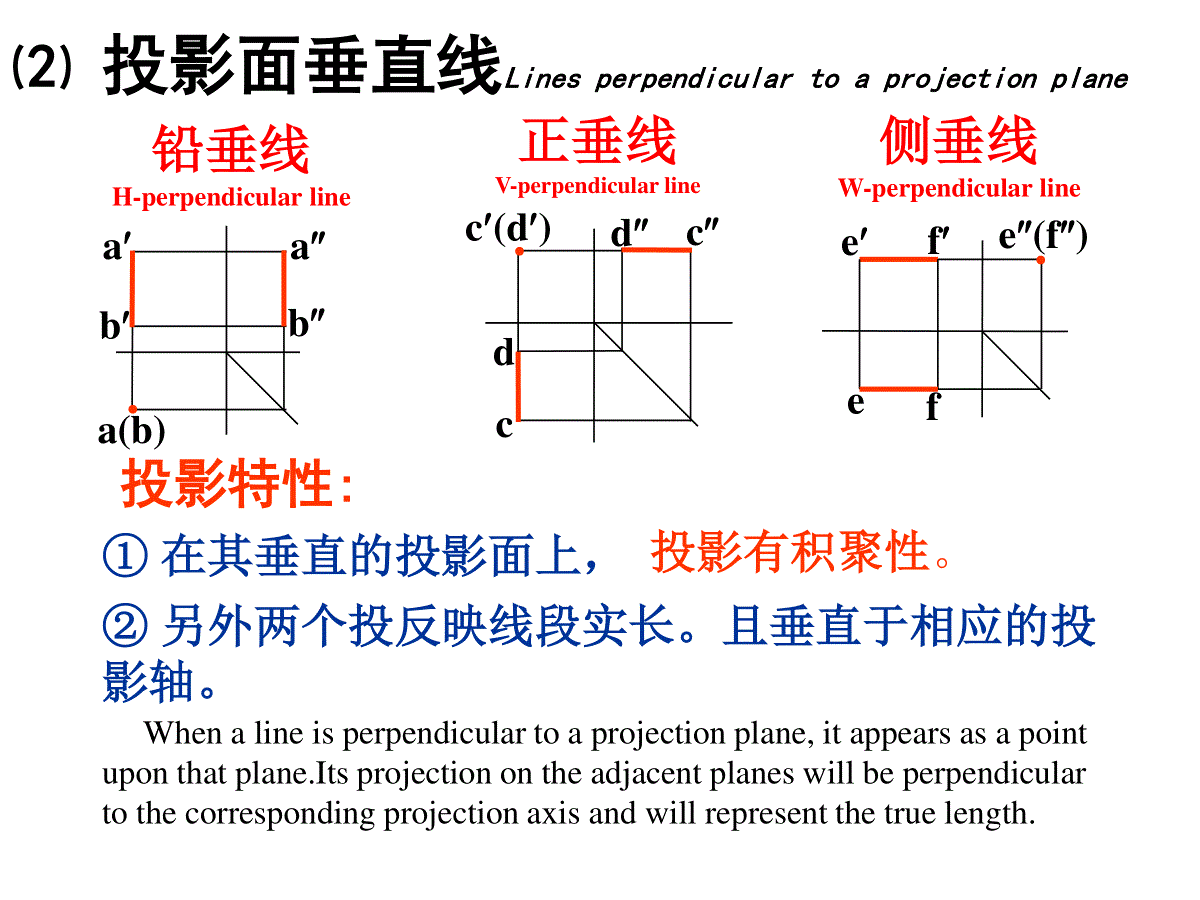
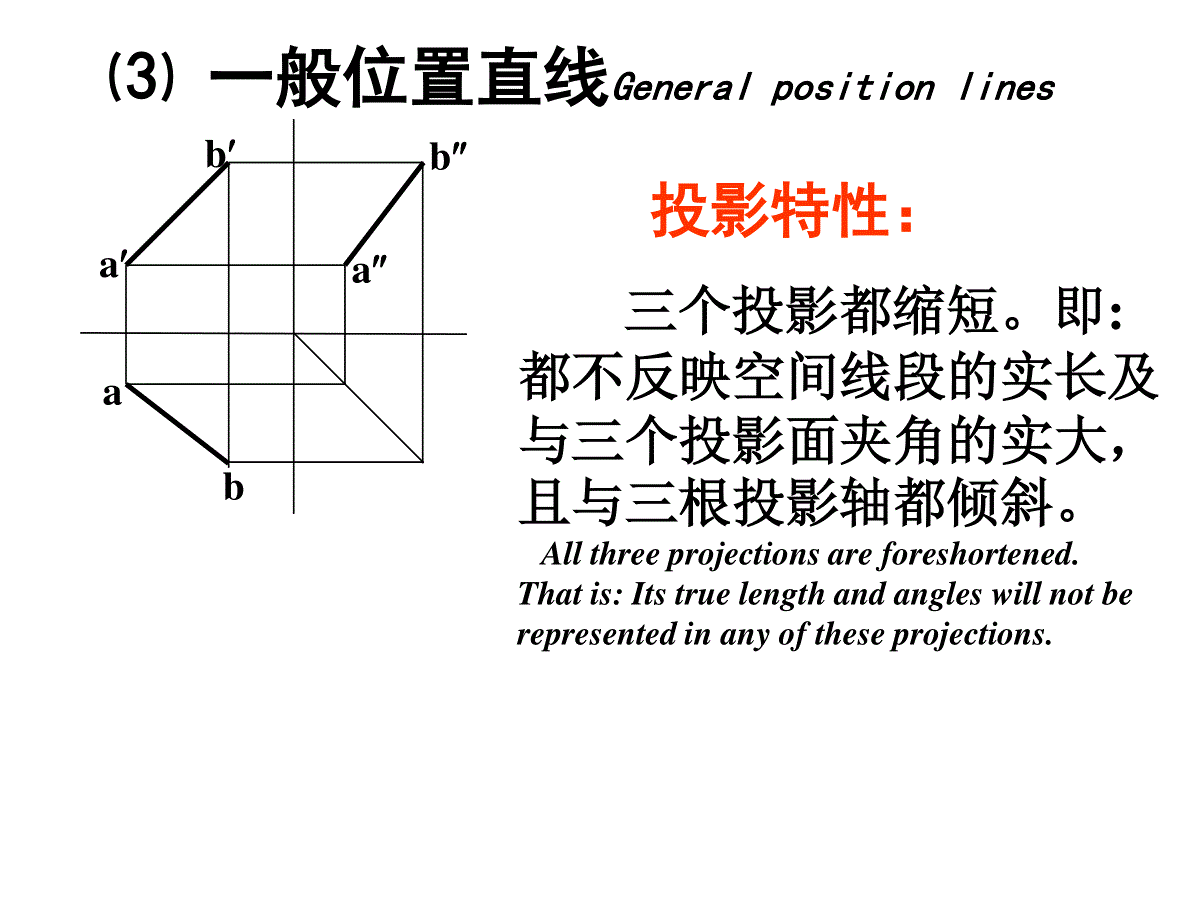
aaabbb●●●●●●2·2直线的投影ProjectionofLine两点确定一条直线，将两点的同名投影用直线连接，就得到直线的同名投影。(Projectionsof2pointsdeterminetheprojectionofline.)⒈直线对一个投影面的投影特性(Tosingleprojectionplane)一、直线的投影特性CharacteristicsAB●●●●ab直线垂直于投影面投影重合为一点积聚性Point-wiseprojection直线平行于投影面投影反映线段实长存真性ab=ABTruelengthprojection直线倾斜于投影面投影比空间线段短ab=ABcosαForeshortenprojection●●AB●●abαAMB●a≡b≡m●●●二、直线在三个投影面中的投影特性Linesprojectedtothe3-projection-plane投影面平行线LinesparalleltoaProjectionplane平行于某一投影面而与其余两投影面倾斜投影面垂直线Linesperpendiculartoaprojectionplane正平线Frontallines（∥Ｖ面）侧平线Profilelines（∥Ｗ面）水平线Horizontallines（∥Ｈ面）正垂线（W-perpendicularlines）（⊥Ｖ面）侧垂线（W-perpendicularlines）（⊥Ｗ面）铅垂线（H-perpendicularlines）（⊥Ｈ面）一般位置直线GeneralPositionlines与三个投影面都倾斜的直线Inclinedtoallthreeprojectionplanes统称特殊位置直线(Specialpositionlines)垂直于某一投影面baababbaabba⑴投影面平行线LinesparalleltoaProjectionplane①在其平行的那个投影面上的投影反映实长，并反映直线与另两投影面倾角的实大。②另两个投影面上的投影平行于相应的投影轴。Whenalineisparalleltoaprojectionplane,itsprojectionuponthatplanewillbethetruelengthoftheline.Itsprojectionontheadjacentplaneswillbeparalleltotheprojectionaxis.水平线Horizontalline侧平线Profileline正平线Frontallineγ投影特性：与H面的夹角（AnglewithH）:α与V面的角:β与W面的夹角:γ实长（Truelength）实长实长βγααβbaaabb⑵投影面垂直线Linesperpendiculartoaprojectionplane铅垂线H-perpendicularline正垂线V-perpendicularline侧垂线W-perpendicularline②另外两个投反映线段实长。且垂直于相应的投影轴。①在其垂直的投影面上，投影有积聚性。投影特性:●c(d)cddc●aba(b)ab●efefe(f)Whenalineisperpendiculartoaprojectionplane,itappearsasapointuponthatplane.Itsprojectionontheadjacentplaneswillbeperpendiculartothecorrespondingprojectionaxisandwillrepresentthetruelength.⑶一般位置直线Generalpositionlines投影特性：三个投影都缩短。即:都不反映空间线段的实长及与三个投影面夹角的实大，且与三根投影轴都倾斜。Allthreeprojectionsareforeshortened.Thatis:Itstruelengthandangleswillnotberepresentedinanyoftheseprojections.abbaba三、直线上的点Pointsonlines◆若点在直线上,则点的投影必在直线的同名投影上。Ifapointinspaceliesonaline,theprojectionsofthatpointalsolieonthecorrespondingprojectionsoftheline.判别方法：ABCVHbccbaa点C不在直线AB上(No)例1：判断点C是否在线段AB上。DetermineifthepointConlineABabcabc①c②abcab●点C在直线AB上(Yes)例2：判断点K是否在线段AB上。DetermineifthepointKonlineABab●k因k不在ab上，故点K不在AB上。kisnotonab,thenKliesonlineAB.abkabk●●◆当直线的投影垂直于投影轴时有例外出现，这种情况可增加第三投影。Exceptionmayoccurtospecialpositionline.Aprofileprojectionisaddedinthiscase.四、一般位置直线的实长与倾角TruelengthandangleofagenerallineOXHVaaaxb`bbx倾角:直线与在某一投影面上的投影间的夹角为直线与该面的倾角Anglebetweenlineanditsprojectionwillbeitsanglewiththatprojectionplane.OXYZHVaAaaxb`BbbxOXYZHVa`AaWa``b`Bbb``abAH投影立标差⊿Zαα直角三角形法RightTriangleMethodabAH投影立标差⊿ZαabAV投影远标差⊿YβabAW投影横标差⊿Xγ斜边（Beveledge）--实长（Truelength）斜边与直角边夹角--倾角Anglebetweenthebeveledgeandright-angleedge(Angle)规律（Rule）：两直角边（tworight-angleedges）投影(Projection)坐标差(Coordinatedifference)直线与投影轴的夹角(Anglebetweenlineandprojectionaxis)OXYZHVaAaWabBbbabAH投影立标差⊿ZααZZabAH投影立标差⊿ZαabAV投影远标差⊿YβabAW投影横标差⊿Xγ斜边（Beveledge）--实长（Truelength）斜边与直角边夹角--倾角Anglebetweenthebeveledgeandright-angleedge--Anglebetweenlineandplane)规律（Rule）：两直角边（tworight-angleedges）投影(Projection)坐标差(Coordinatedifference)斜边与另一直角边夹角--直线与投影轴夹角Anglebetweenthebeveledgeandtheotherright-angleedge--AnglebetweenlineandaxisZYX3.作图举例(Problems)例1.已知直线段AB与H面的倾角α=30º,其他条件如图,完成AB的另一投影。CompletetheotherprojectionoflineABwithα=30ºa`b`abα=30ºZB1例2.已知直线段AB与H面的倾角α=30º,其他条件如图,完成AB的另一投影。CompletetheotherprojectionoflineABwithα=30ºa`b`abα=30ºZA1H投影b1α实长Z空间两直线的相对位置分为：平行Parallel、相交intersecting、交叉skew。⒈两直线平行Parallellines投影特性：Projectioncharacteristics空间两直线平行，则其各同名投影必相互平行，反之亦然。Iftwolinesinspaceareparallel,theirprojectionsonanyprojectionplanewillbeparallel,andviceversa.aVHcbcdABCDbda五、空间两直线的相对位置Relativepositionoftwolinesabcdcabd例1：判断图中两条直线是否平行。ABParallelwithCD?对于一般位置直线，只要有两个同名投影互相平行，空间两直线就平行。Twogenerallinesareparallelinspaceiftheirrespectiveprojectionsontwoprojectionplanesareparallel.AB//CD①bdcacbaddbac对于特殊位置直线，只有两个同名投影互相平行，空间直线不一定平行。Forspecialpositionlines,theyarenotnecessarilyparallelifonlytwoprojectionsappeartobeparallel.求出侧面投影后可知：AB与CD不平行。ABandCDnotparallel例2：判断图中两条直线是否平行。ABParallelwithCD?②求出侧面投影Obtaintheprofileprojection如何判断？Sohowto?HVABCDKabcdkabckdabcdbacdkk⒉两直线相交Intersectinglines判别方法：若空间两直线相交，则其同名投影必相交，且交点的投影必符合空间一点的投影规律。Iftwolinesintersectinspace,theircorrespondingprojectionsalsointersect.交点是两直线的共有点(intersectingpointistheonewhichtwolinesshare)●●cabbacdkkd例：过C点作水平线CD与AB相交。ConstructahorizontallineCDintersectingwithAB.先作正面投影Vprojectionfirstdbaabcdc1(2)3(4)⒊两直线交叉Skewlines投影特性Projectioncharacteristics★同名投影可能相交，但“交点”不符合空间一个点的投影规律。Correspondingprojectionsmaybeintersecting,but“intersectingpoints”don’tabidebytheprojectionruleofonespatialpoint.★“交点”是两直线上的一对重影点的投影，用其可帮助判断两直线的空间位置。“intersectingpoints”aretheprojectionsofthetwooverlappedprojectionpoints,whichcanbeusedforjudgingthespatialpositionsoftwolines.●●Ⅰ、Ⅱ是Ｖ面的重影点(Overlappedprojectionpoints)Ⅲ、Ⅳ是H面的重影点为什么？Why?12●●34●●两直线相交吗？Intersectinglines?六、两直线垂直相交(或交叉）Perpendicularityoflines直角边投影的规律：Thetheoremofperpendicularity若直角有一边平行于投影面，则它在该投影面上的投影仍为直角。Ifoneoftwoperpendicularlinesisparalleltoaprojectionplane,theprojectionsofbothlinesonthatplanewillbeat90ºtoeachother.设直角边BC//H面因BC