Signal-and-system-test-paper-2009-11

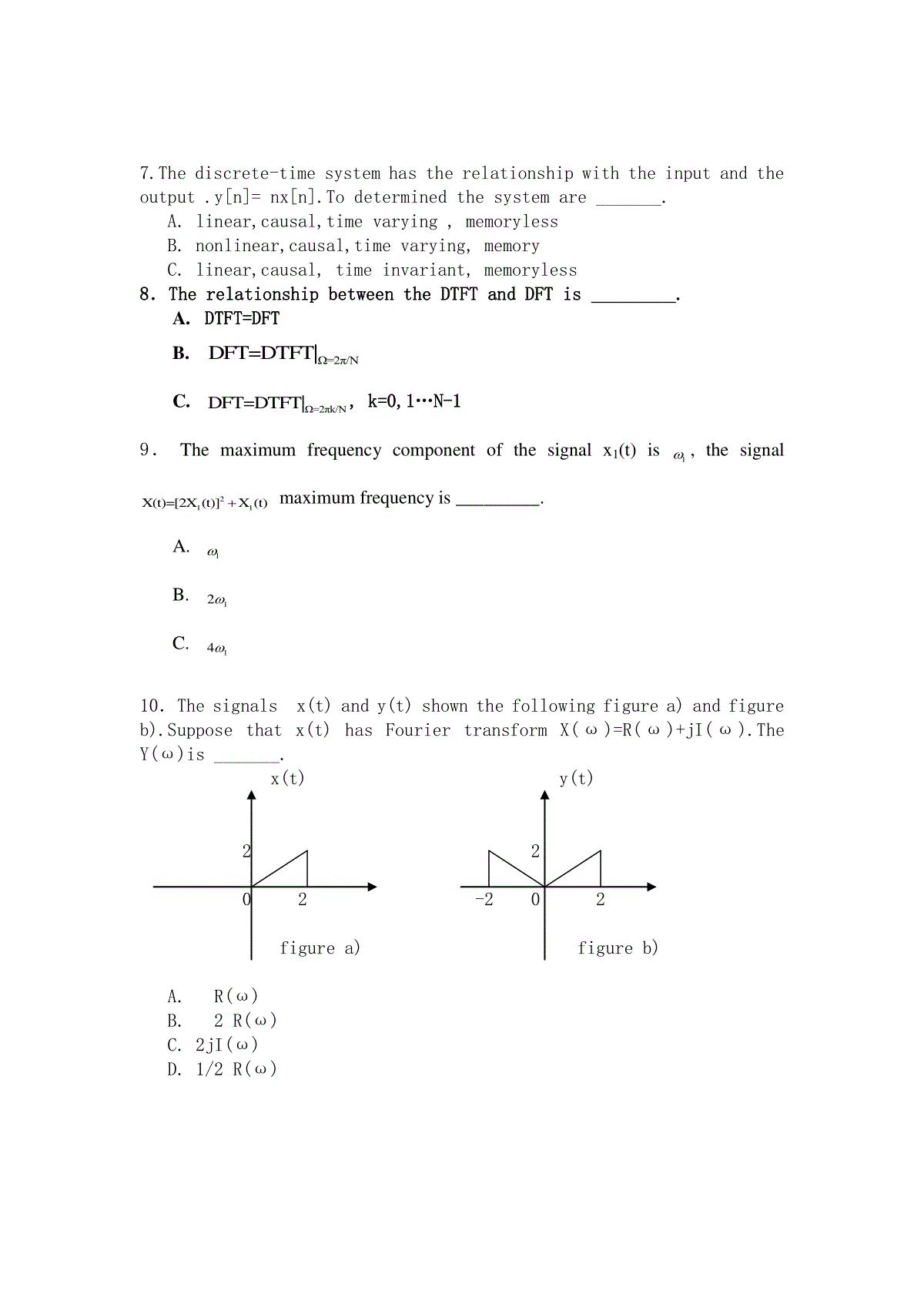
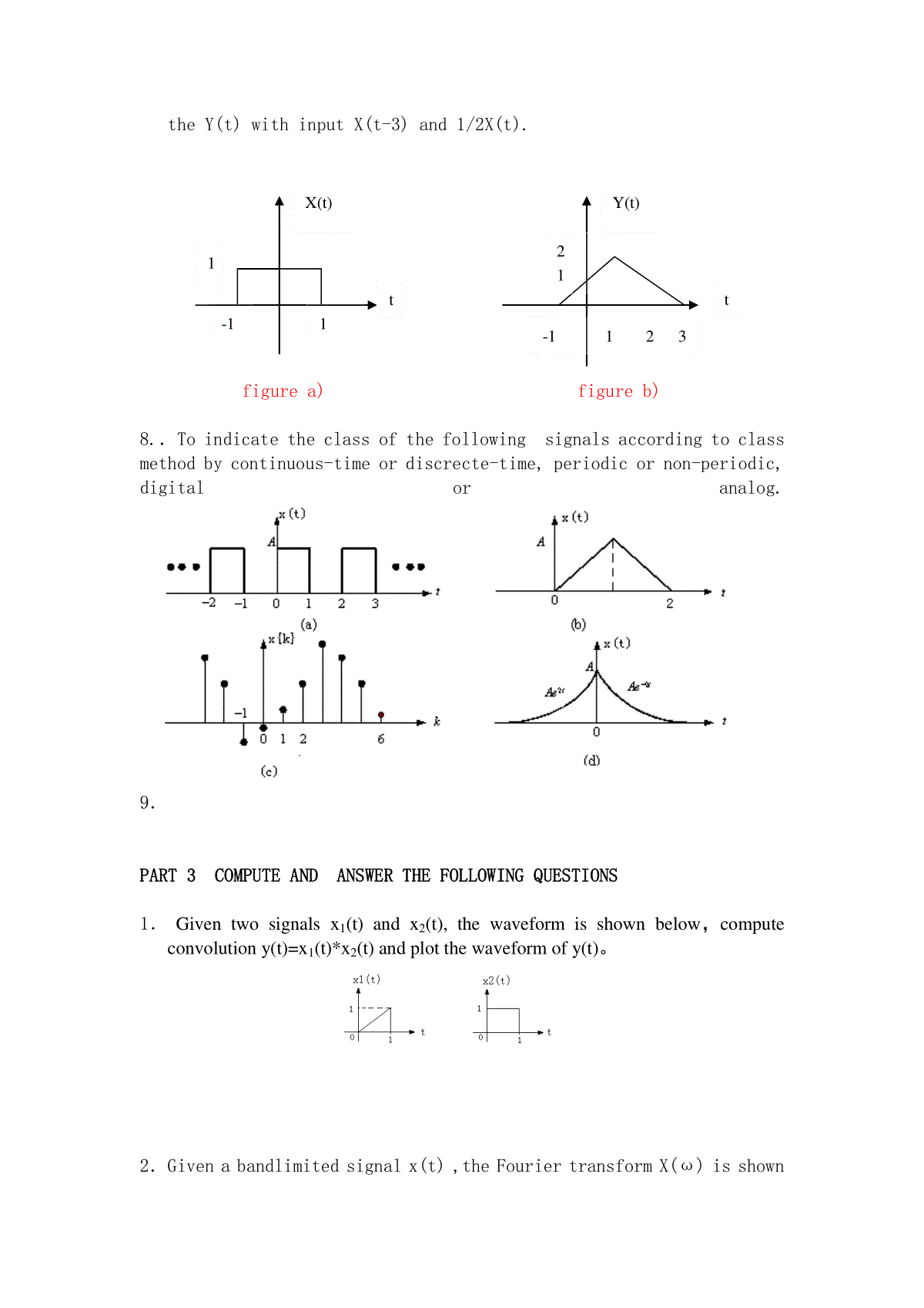
Signalandsystemtestpaper2011-10-22ForElectricalEngineeringDepartment2009PART1CHOOSINGTHEBESTANSWER1.Thefollowingsignalsareshownbelow,the______isperiodicsignal.A.x(t)=costu(t)B.x[n]=cos(1/4)nC.X(Ω)=TheFouriertransformofx[n]2.TheFouriertransformofperiodicsignalxT(t)is_______.A.discretevaluesckB.impulsetrain（冲激序列）C.continuousfunction3.Thezerostateresponseofastablelineartimeinvariantsystemisshownbelow,theinputsignalisprobably_________.A.stepsignalB.impulsesignalC.sinusoidalsignal4.Theinput/outputrelationshipofthediscrete-timesystemintimedomainis______.D.TheFouriertransformrepresentationandtheconvolutionmodel.E.Theinput/outputdifferentialequationandtheconvolutionmodel.F.Theinput/outputdifferenceequationandtheconvolutionmodel.5.TherectangularpulsetrainisperiodicsignalwithfundamentalperiodTheamplitudespectrumis|Ck|.WhenTbecomeslarge,Then________A.|Ck|becomeslargerandthelinespectruminterval(谱线间隔)becomeslargerB.|Ck|becomessmallerandthelinespectrumintervalbecomeslargerC.|Ck|becomessmallerandthelinespectrumintervalbecomessmaller6.Supposethatx(t)isgivenbyx(t)={x1(t)t1≤t﹤t2andx2(t)t≥t2}.Thesignalx(t)canbeexpressed_______.G.x(t)=x1(t)[u(t-t1)-u(t-t2)]+x2(t)[u(t-t1)-u(t-t2)]H.x(t)=x1(t)[u(t-t1)-u(t-t2)]+x2(t)u(t-t2)I.x(t)=[x1(t)+x2(t)][u(t-t1)-u(t-t2)]7.Thediscrete-timesystemhastherelationshipwiththeinputandtheoutput.y[n]=nx[n].Todeterminedthesystemare_______.A.linear,causal,timevarying,memorylessB.nonlinear,causal,timevarying,memoryC.linear,causal,timeinvariant,memoryless8．TherelationshipbetweentheDTFTandDFTis_________.A.DTFT=DFTB.Ω=2π/NDFT=DTFT|C.Ω=2πk/NDFT=DTFT|,k=0,1…N-19．Themaximumfrequencycomponentofthesignalx1(t)is1,thesignal211X(t)=[2X(t)]X(t)maximumfrequencyis_________.A.1B.12C.1410．Thesignalsx(t)andy(t)shownthefollowingfigurea)andfigureb).Supposethatx(t)hasFouriertransformX(ω)=R(ω)+jI(ω).TheY(ω)is_______.x(t)y(t)2202-202figurea)figureb)A.R(ω)B.2R(ω)C.2jI(ω)D.1/2R(ω)PART2ANSWERTHEFOLLOWINGQUESTIONS1.Computethevaluesofthefollowingfunctions:2.Computethevaluesofthefollowingfunctions:a.()dxtdt:(where31()(1)(1)(1)(3)(3)22xttuttuttut)b.()dxtdt:(where()(cos)()xttut)c.121(24)(1)ttdtd.()*()utut3.Givenasystemy(t)=x2(t),determinewhetherthecontinuous-timesystemiscausalornoncausal,hasmemoryorismemeoryless,islinearornonlinear,istimeinvariantortimevarying.Justifyyouranswer.4.Givenaanalogsignalx(t),theFouriertransformisX(ω),computetheFouriertransformofsignalx(2t-5).5.ALTIsystem,underthesameinitialconditions,whentheinputsignalisx(t),thecompleteresponseis31()(2sin2)()tytetut,whentheinputsignalis2x(t),thecompleteresponseis32()(2sin2)()tytetut;computethecompleteresponseforthefollowingsignalsunderthesameinitialcondition.a.0()xttb.0.5()xt6.Givenasignalx(t),theFouriertransformX(ω)isshownbelow,thesampledsignalisxs(t)=x(t)p(t),theFouriertransformofxs(t)isXs(ω),plotthe|Xs(ω)|,assumethesamplefrequencyωs2B.7.Givenalineartime-invariantcontinuous-timesystem.TheinputX(t)andoutputY(t)shownthefollowingfigurea)andfigureb).Plot01X(ω)ω-BBtheY(t)withinputX(t-3)and1/2X(t).figurea)figureb)8.．Toindicatetheclassofthefollowingsignalsaccordingtoclassmethodbycontinuous-timeordiscrecte-time,periodicornon-periodic,digitaloranalog.9．PART3COMPUTEANDANSWERTHEFOLLOWINGQUESTIONS1．Giventwosignalsx1(t)andx2(t),thewaveformisshownbelow，computeconvolutiony(t)=x1(t)*x2(t)andplotthewaveformofy(t)。2．Givenabandlimitedsignalx(t),theFouriertransformX(ω)isshownX(t)t11Y(t)t-112321-1inFig.A.ThesystemisshowninFig.B.X(ω)x(t)a(t)b(t)××-10010cos30tcos10tFigAFigBPlottheA(ω),B(ω)3.Giventwosignalsx1(t)=x2(t)=computetheconvolutiony(t)=x1(t)*x2(t)andtheFouriertransformY(ω),plotthewaveformofy(t)and|Y(ω)|.4．GiventhefrequencyresponsefunctionoftheideallowpassfilterH(ω)={1,|ω|ωcand0,|ω|ωc}.theinputsignalx(t)=sinat/Пt.Computeandanalysethefollowingquestions.(a).ifaωc,thefilteroutputy(t).(b).ifaωc,thefilteroutputy(t).(c).whichcasecomeintobeingtheoutputdistortion.(wherex(t)=sinat/Пt---取样函数)5.Giventhesystemisshownfollowingthefigure.Theinputsin()tfttAndnsTnTtt),()(Ts=0.5s.（---productintimedomain）1).ComputefA(t),FA(ω)andplotthe|FA(ω)|.2)Computey(t),y(ω)andplotthe|y(ω)|.()Tty(t)fA(t)f(t)2121,1,0{ttotherallSolution(1)()Sa()()()()fttGjuu2()()()Sa()Aftftftt又)]2()()[2(21)]()2()[2(21)(*)(21)(uuuujFjFjFA1(2)[(2)(2)]2uu(2)12()[()]2[(4)]AAnnssnYjFjFjnTTFA(j)221…222Y(j)…4-4