统计学第五章

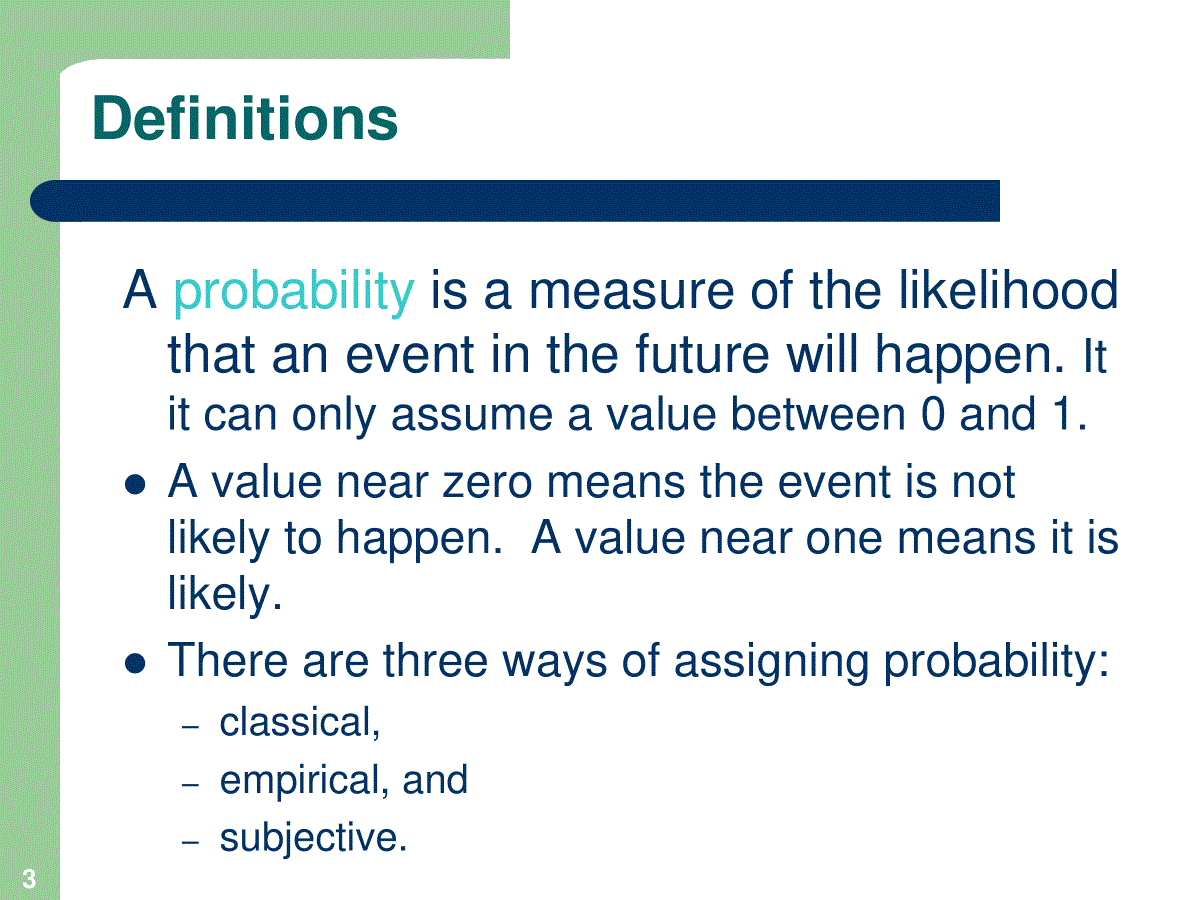
©TheMcGraw-HillCompanies,Inc.2008McGraw-Hill/IrwinASurveyofProbabilityConceptsChapter52GOALSDefineprobability.Describetheclassical,empirical,andsubjectiveapproachestoprobability.Explainthetermsexperiment,event,outcome,permutations,andcombinations.Definethetermsconditionalprobabilityandjointprobability.Calculateprobabilitiesusingtherulesofadditionandrulesofmultiplication.Applyatreediagramtoorganizeandcomputeprobabilities.CalculateaprobabilityusingBayes’theorem.3DefinitionsAprobabilityisameasureofthelikelihoodthataneventinthefuturewillhappen.Ititcanonlyassumeavaluebetween0and1.Avaluenearzeromeanstheeventisnotlikelytohappen.Avaluenearonemeansitislikely.Therearethreewaysofassigningprobability:–classical,–empirical,and–subjective.4ProbabilityExamples5DefinitionscontinuedAnexperimentistheobservationofsomeactivityortheactoftakingsomemeasurement.Anoutcomeistheparticularresultofanexperiment.Aneventisthecollectionofoneormoreoutcomesofanexperiment.6Experiments,EventsandOutcomes7AssigningProbabilitiesThreeapproachestoassigningprobabilities–Classical–Empirical–Subjective8ClassicalProbabilityConsideranexperimentofrollingasix-sideddie.Whatistheprobabilityoftheevent“anevennumberofspotsappearfaceup”?Thepossibleoutcomesare:Therearethree“favorable”outcomes(atwo,afour,andasix)inthecollectionofsixequallylikelypossibleoutcomes.9MutuallyExclusiveEventsEventsaremutuallyexclusiveiftheoccurrenceofanyoneeventmeansthatnoneoftheotherscanoccuratthesametime.Eventsareindependentiftheoccurrenceofoneeventdoesnotaffecttheoccurrenceofanother.10CollectivelyExhaustiveEventsEventsarecollectivelyexhaustiveifatleastoneoftheeventsmustoccurwhenanexperimentisconducted.11EmpiricalProbabilityTheempiricalapproachtoprobabilityisbasedonwhatiscalledthelawoflargenumbers.Thekeytoestablishingprobabilitiesempiricallyisthatmoreobservationswillprovideamoreaccurateestimateoftheprobability.12LawofLargeNumbersSupposewetossafaircoin.Theresultofeachtossiseitheraheadoratail.Ifwetossthecoinagreatnumberoftimes,theprobabilityoftheoutcomeofheadswillapproach.5.Thefollowingtablereportstheresultsofanexperimentofflippingafaircoin1,10,50,100,500,1,000and10,000timesandthencomputingtherelativefrequencyofheads13EmpiricalProbability-ExampleOnFebruary1,2003,theSpaceShuttleColumbiaexploded.Thiswastheseconddisasterin113spacemissionsforNASA.Onthebasisofthisinformation,whatistheprobabilitythatafuturemissionissuccessfullycompleted?98.0113111flightsofnumberTotalflightssuccessfulofNumberflightsuccessfulaofyProbabilit14SubjectiveProbability-ExampleIfthereislittleornopastexperienceorinformationonwhichtobaseaprobability,itmaybearrivedatsubjectively.Illustrationsofsubjectiveprobabilityare:1.EstimatingthelikelihoodtheNewEnglandPatriotswillplayintheSuperBowlnextyear.2.Estimatingthelikelihoodyouwillbemarriedbeforetheageof30.3.EstimatingthelikelihoodtheU.S.budgetdeficitwillbereducedbyhalfinthenext10years.15SummaryofTypesofProbability16RulesforComputingProbabilitiesRulesofAdditionSpecialRuleofAddition-IftwoeventsAandBaremutuallyexclusive,theprobabilityofoneortheotherevent’soccurringequalsthesumoftheirprobabilities.P(AorB)=P(A)+P(B)TheGeneralRuleofAddition-IfAandBaretwoeventsthatarenotmutuallyexclusive,thenP(AorB)isgivenbythefollowingformula:P(AorB)=P(A)+P(B)-P(AandB)17AdditionRule-ExampleWhatistheprobabilitythatacardchosenatrandomfromastandarddeckofcardswillbeeitherakingoraheart?P(AorB)=P(A)+P(B)-P(AandB)=4/52+13/52-1/52=16/52,or.307718TheComplementRuleThecomplementruleisusedtodeterminetheprobabilityofaneventoccurringbysubtractingtheprobabilityoftheeventnotoccurringfrom1.P(A)+P(~A)=1orP(A)=1-P(~A).19JointProbability–VennDiagramJOINTPROBABILITYAprobabilitythatmeasuresthelikelihoodtwoormoreeventswillhappenconcurrently.20SpecialRuleofMultiplicationThespecialruleofmultiplicationrequiresthattwoeventsAandBareindependent.TwoeventsAandBareindependentiftheoccurrenceofonehasnoeffectontheprobabilityoftheoccurrenceoftheother.Thisruleiswritten:P(AandB)=P(A)P(B)21MultiplicationRule-ExampleAsurveybytheAmericanAutomobileassociation(AAA)revealed60percentofitsmembersmadeairlinereservationslastyear.Twomembersareselectedatrandom.Whatistheprobabilitybothmadeairlinereservationslastyear?Solution:Theprobabilitythefirstmembermadeanairlinereservationlastyearis.60,writtenasP(R1)=.60Theprobabilitythatthesecondmemberselectedmadeareservationisalso.60,soP(R2)=.60.SincethenumberofAAAmembersisverylarge,youmayassumethatR1andR2areindependent.P(R1andR2)=P(R1)P(R2)=(.60)(.60)=.3622ConditionalProbabilityAconditionalprobabilityistheprobabilityofaparticulareventoccurring,giventhatanothereventhasoccurred.TheprobabilityoftheeventAgiventhattheeventBhasoccurrediswrittenP(A|B).23GeneralMultiplicationRuleThegeneralruleofmultiplicationisusedtofindthejointprobabilitythattwoeventswilloccur.Usethegeneralruleofmultiplicationtofindthejointprobabilityoftwoeventswhentheeventsarenotindependent.Itstatesthatfortwoevents,AandB,thejointprobabilitythatbotheventswillhappenisfoundbymultiplyingtheprobabilitythateventAwillhappenbytheco