当前位置:首页 > 商业/管理/HR > 信息化管理 > 投资学第5章利率史与风险溢价1student
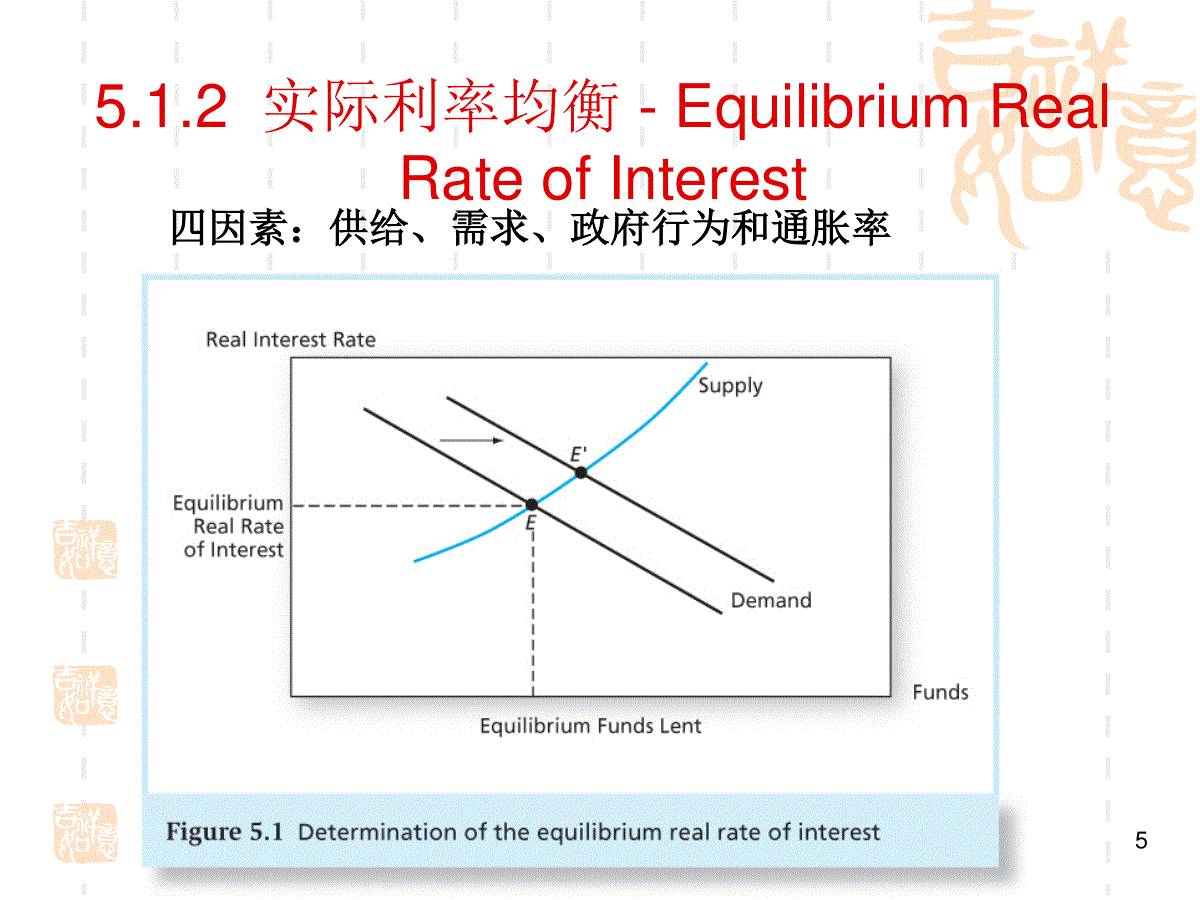
投资学第5章历史数据中的收益与风险IntroductiontoRisk,Return,andtheHistoricalRecord2本章主要内容利率水平的确定-InterestRateDeterminants期望收益与波动性–ExpectedReturnanditsVariance风险价值–ValueatRisk35.1利率水平的确定利率水平的决定因素:资金供给(居民)-Households资金需求(企业)-Businesses资金供求的外生影响(政府)-Government’sNetSupplyand/orDemandFederalReserveActions45.1.1实际利率(realinterestrate)与名义利率(nominalinterestrate)消费者物价指数(CPI,consumerpriceindex)Nominalinterestrate(R):GrowthrateofyourmoneyRealinterestrate(r):GrowthrateofyourpurchasingpoweriiRriRriRriRr1111,严格上讲,有:为通胀率为名义利率,为实际利率,其中近似地看,有:55.1.2实际利率均衡-EquilibriumRealRateofInterest四因素:供给、需求、政府行为和通胀率●●资金均衡资金借出均衡的真实利率利率E’E需求供给利率均衡的真实利率利率均衡资金借出均衡的真实利率利率资金均衡资金借出均衡的真实利率利率供给资金均衡资金借出均衡的真实利率利率65.1.3名义利率均衡-EquilibriumNominalRateofInterest费雪方程(Fisherequation)含义:名义利率应该随预期通胀率的增加而增加Astheinflationrateincreases,investorswilldemandhighernominalratesofreturnIfE(i)denotescurrentexpectationsofinflation,thenwegettheFisherEquation:Nominalrate=realrate+inflationforecast)(iErR75.1.4税收与实际利率着通胀率的上升而下降可见:税后实际利率随税后真实利率为:则税后名义利率为,,名义利率为记税率为ittritiritRtRRt)1()1)(()1()1(85.2持有期收益率1/Tf(T)r1EAR1rate)annualeffective(EAR,1)(100)()(,则为:折为有效年利率益率为:则贴现债券的持有期收为买入价格,为持有期,若TPTrTPTfZeroCouponBond,Par=$100,T=maturity,P=price,rf(T)=totalriskfreereturn5-9Example5.2AnnualizedRatesofReturn5-10Equation5.7实际年利率-EAREffectiveannualratedefinition:percentageincreaseinfundsinvestedovera1-yearhorizonTfTrEAR111115.2.1年百分比利率TEARAPRAPRTTrTrEARAPRTTrTrnAPRTrTTTfnffff1)1(即:1)(1)(11更一般地,有:)(或)(,则有:)(期,每期利率为T1n一年为来表示,即若rate)percentageannual,(APR分比利率短期投资利率常用年百/1/15-12Equation5.8年百分比率-APRTEARAPRT11TfTrEAR1115-13Table5.1APRvs.EAR145.2.2连续复利收益率当T趋于无限小时,可得连续复利(continuouscompounding)概念)1ln(11lim1/10EARreEAReAPRTEARccrrTTcccc即:5-15Table5.2StatisticsforT-BillRates,InflationRatesandRealRates,1926-20095-16Figure5.3InterestRatesandInflation,1926-200917Figure5.4NominalandRealWealthIndexesforInvestmentinTreasuryBills,1966-2005185.4风险和风险溢价riskpremium5.4.1持有期收益holdingperiodreturn股票收益包括两部分:红利收益(dividends)与资本利得(capitalgains)持有期收益率(holding-periodreturn)期初价格现金红利期初价格股票期末价格-HPR5-19RiskandRiskPremiumsPDPPHPR0101HPR=HoldingPeriodReturnP0=BeginningpriceP1=EndingpriceD1=DividendduringperiodoneRatesofReturn:SinglePeriod5-20EndingPrice=110BeginningPrice=100Dividend=4HPR=(110-100+4)/(100)=14%RatesofReturn:SinglePeriodExample215.4.2期望收益expectedreturn与标准差standarddeviation:E-V方法WearenotsureabouttheeventualHPR,sowehavetoknowtheProbabilityDistributionofthefutureoutcome.WewillcharacterizePDintermsoftheirexpectedreturnE(r)andtheirstandarddeviationσ.22)]()([)()()()()()()(rEsrspsrsprErEHPRsrspsss则有:为标准差为期望收益,,为各情形的为各情形的概率,,记不确定情形的集合为5-22StateProb.ofStaterinStateExcellent.250.3100Good.450.1400Poor.25-0.0675Crash.05-0.5200E(r)=(.25)(.31)+(.45)(.14)+(.25)(-.0675)+(0.05)(-0.52)E(r)=.0976or9.76%ScenarioReturns:Example5-23Variance(VAR):VarianceandStandardDeviation22()()()spsrsEr2STDStandardDeviation(STD):5-24ScenarioVARandSTDExampleVARcalculation:σ2=.25(.31-0.0976)2+.45(.14-.0976)2+.25(-0.0675-0.0976)2+.05(-.52-.0976)2=.038ExampleSTDcalculation:1949.038.25例:假定投资于某股票,初始价格100美元,持有期1年,现金红利为4美元,预期股票价格由如下三种可能,求其期望收益和方差。(1)(1401004)/10044%r26σ=450^0.5=21.2132275.4.3超额收益与风险溢价–RiskandRiskpremiumsExample:rf=6%,rstockA=14%,sowhatis8%whichequalstorstockA-rf?rstockA-rf=excessreturn,orexcessreturn=actualreturn–riskfreerate.Theriskpremiumistheexpectedvalueoftheexcessreturn,thenE(r)-rf=riskpremium.WemeasurethereturnofaninvestmentwithitsE(r),wemeasuretheriskofaninvestmentwithitsriskpremium’sstandarddeviation.285.4.3超额收益与风险溢价–RiskandRiskpremiums例:上例中我们得到股票的预期回报率E(r)为14%,若无风险收益率为rf8%。初始投资100元于股票,其风险溢价(E(r)-rf)为6元,作为其承担风险(标准差为21.2元)的补偿。投资者对风险资产投资的满意度取决于其风险厌恶(riskaversion)程度295.5历史收益率时间序列分析5.5.1时间序列与情景分析WedonotknowthePDoffutureoutcomes,aswellastheirE(r)andσ.Wemustinferfromitshistoryortimeseriesinordertoestimatethem.5.5.2期望收益与算术平均收益率的算术平均数arithmeticaverageofratesofreturn:nsnssrnsrsprE11)(1)()()(305.5.2几何收益率GeometricAverageReturnTV=投资终值(TerminalValueoftheInvestment)1/1TVgng=几何平均收益率(geometricaveragerateofreturn))1()1)(1(21nnrrrTV315.5.4方差与标准差方差=期望值偏离的平方(expectedvalueofsquareddeviations)历史数据的方差估计:𝜎2=𝑝(2)𝑟𝑠−𝐸(𝑟)2𝜎2=1𝑛𝑟𝑠−𝑟2𝑛𝑠=1无偏化处理:𝜎=1𝑛−1𝑟𝑠−𝑟2𝑛𝑠=1325.5.5报酬-风险比率(夏普比率)TheReward-to-Volatility(Sharpe)RatioSharpeRatioforPortfolios=RiskPremiumSDofExcessReturnWewouldliketoknowthetrade-offbetweenreward(theriskpremium)andrisk(asmeasuredbystandarddeviationorSD)5-335.6正态分布-TheNormalDistributionInvestmentmanagementiseasierwhenreturnsarenormal.Standarddeviationisagoodmeasureofriskwhenreturnsaresymmetric.Ifsecurityreturnsaresymmetric,portfolioreturnswillbe,too.Futurescenarioscanbeestimatedusingonlythemeanandthestandarddeviation.345.6正态分布-TheNormalDistribution5-35NormalityandRiskMeasuresWhatifexcessreturnsarenotnormallydistributed?StandarddeviationisnolongeracompletemeasureofriskSharperatioisnotacompletemeasureofportfolioperformanceNeedtoconsiderskewandkurt




 三七文档所有资源均是用户自行上传分享,仅供网友学习交流,未经上传用户书面授权,请勿作他用。
三七文档所有资源均是用户自行上传分享,仅供网友学习交流,未经上传用户书面授权,请勿作他用。
本文标题:投资学第5章利率史与风险溢价1student
链接地址:https://www.777doc.com/doc-520997 .html