当前位置:首页 > 金融/证券 > 综合/其它 > 合肥工业大学高数习题册上册答案详解
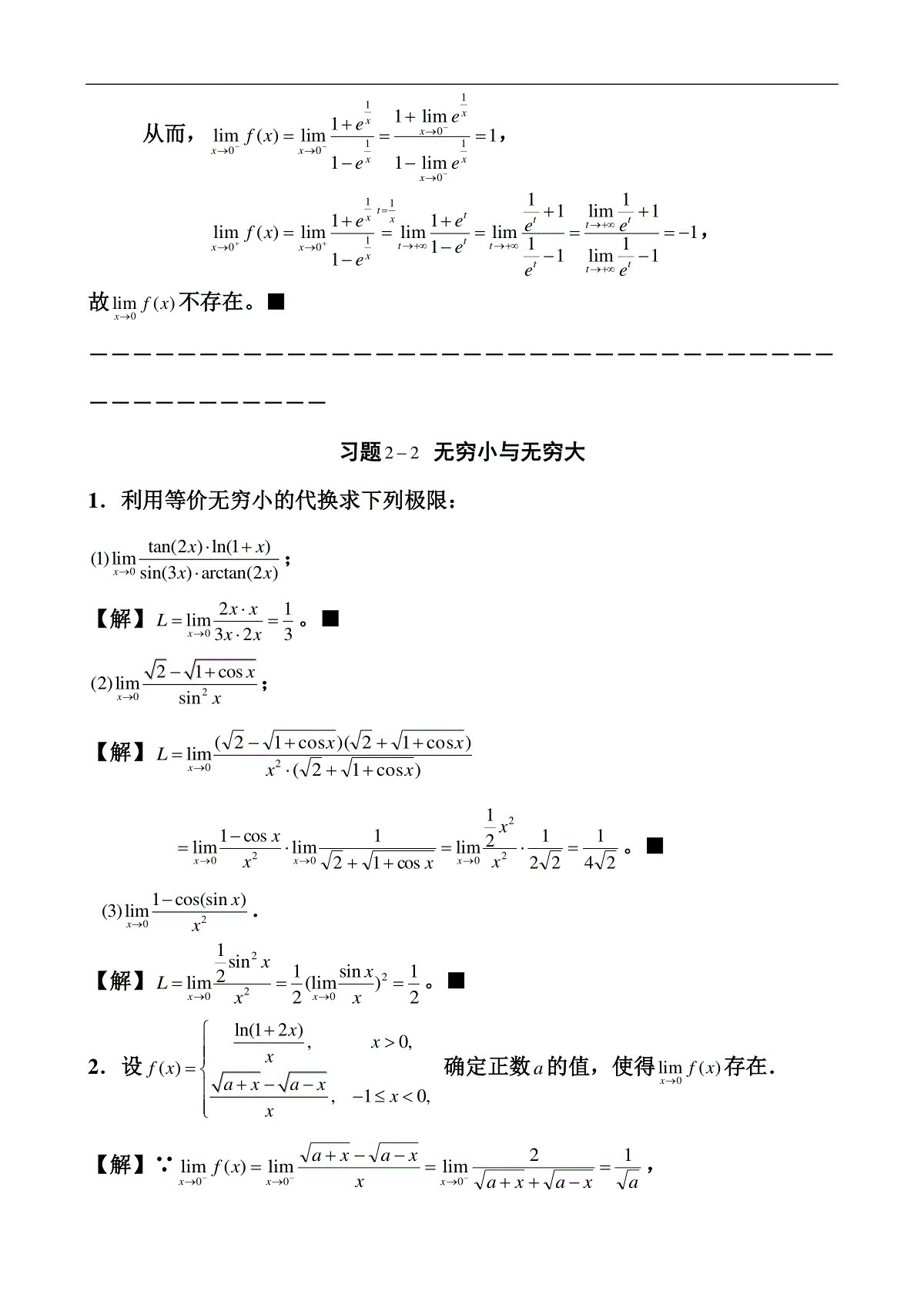
习题11函数1.设函数2,0,()2,0,xxxfxx,求(1)(1)f,(0)f,(1)f;(2)()(0)fxfx,()(0)fxfx(0x).【解】(1)2|2)1(,2|)2()0(,1|)2()1(101xxxxfxfxf;(2)()(0)fxfx.0,1,0,220,2)2(,0,22xxxxxxxxxx()(0)fxfx)0(12)2(xxx。■2.已知21()1fxxx,求()fx.【解】令xt1,则2111)(tttf,故2111)(xxxf。■3.证明:()2sinfxxx在(,)内是严格递增函数.【证】方法1(定义法)∵对任意2121),,(,xxxx,有)sin2()sin2()()(112212xxxxxfxf2sin2cos2)(2sinsin)(21221121212xxxxxxxxxx2)1(2)(22sin)1(2)(212121212xxxxxxxx012xx,其中用到)0(sin,cos1xxxx,∴()2sinfxxx在(,)内是严格递增函数。方法2(导数法)∵)(0cos2)(xxxf∴),()(xf。■4.设()fx在[,]aa上是奇函数,证明:若()fx在[0,]a上递增,则()fx在[,0]a上也递增.【证】∵对任意0,],0,[,2121axxaxx,有2121],,0[,xxaxx,∴由()fx在)0](,0[aa上单调增加可得:)()(21xfxf。又∵()fx在[,]aa上是奇函数,即)()(),()(2211xfxfxfxf,∴)()(21xfxf,即)()(21xfxf,故()fx在[,0]a上也是单调增加。■―――――――――――――――――――――――――――――――――――――――――――――习题21极限1.求下列极限:11(2)3(1)lim(2)3nnnnn;【解】分之分母同除n3,利用四则运算极限法则和幂极限可得313)32)(2(1)32(limnnnL。■222111(2)lim(1)(1)(1)23nn;【解】∵)11]()1(11[)411)(311)(211(22222nn22222222221)1(1)1(414313212nnnn22222)1)(1()1()2(453342231nnnnnnnnnn21111111121,∴2121limnnLn。■22(3)lim[(1)(1)(1)]nnrrr(1)r;【解】∵rrrrrrrrnn1)1()1)(1)(1()1()1)(1(2222rrrrrrnn111)1()1)(1(12222,∴rrrrrLnnnn111lim111lim1122。■(4)lim(1)xxxx;【解】∵)1()1)(1()1(xxxxxxxxxx11111xxxx,∴211111limxLx。■3131(5)lim()11xxx.【解】)1)(1()2)(1(lim12lim1)1(3lim21321321xxxxxxxxxxxLxxx13312lim21xxxx。■2.求常数a和b,使得02lim1xaxbx.【解】∵02lim1xaxbx,0lim0xx,∴02)2(lim0bbaxx,即4b。于是,)24()24)(24(lim2lim0000axxaxaxxbaxxx14241lim)24(lim00aaxaaxxaxxx,∴4ba。■3.若111()1xxefxe,求0lim()xfx,0lim()xfx,0lim()xfx.【解】∵xx1lim0,xx1lim0,∴0lim10xxe,xxe10lim。从而,1lim1lim111lim)(lim10101100xxxxxxxxeeeexf,111lim11lim1111lim11lim11lim)(lim11100ttttttttttxtxxxxeeeeeeeexf,故0lim()xfx不存在。■―――――――――――――――――――――――――――――――――――――――――――――习题22无穷小与无穷大1.利用等价无穷小的代换求下列极限:0tan(2)ln(1)(1)limsin(3)arctan(2)xxxxx;【解】31232lim0xxxxLx。■2021cos(2)limsinxxx;【解】)cos12()cos12)(cos12(lim20xxxxLx24122121limcos121limcos1lim220020xxxxxxxx。■201cos(sin)(3)limxxx.【解】21)sinlim(21sin21lim20220xxxxLxx。■2.设ln(12),0,(),10,xxxfxaxaxxx确定正数a的值,使得0lim()xfx存在.【解】∵axaxaxxaxaxfxxx12limlim)(lim000,22lim)21ln(lim)(lim000xxxxxfxxx,∴当21a,即41a时,0lim()xfx存在。■―――――――――――――――――――――――――――――――――――――――――――――习题23极限存在准则1.计算下列极限:30tansin(1)limxxxx;【解】200020cos1limcos1limsinlim)cos1cos1sin(limxxxxxxxxxxLxxxx212111。■22sin(2)(2)lim4xxx;【解】4141121lim2)2sin(lim22xxxLxx。■2(3)lim()xxxx;【解】22222])211(lim[])211[(limexxLxxxx。■2221(4)lim()1xxxx.【解】212222222)11(lim)11(lim1111limeeexxxxLxxxxxx。■2.设110,x16nnxx(1,2,3,)n,试证数列nx的极限存在,并求此数列极限.【证】(1)证明极限的存在性·单调性:∵46,10121xxx,∴010412xx。∵111116666nnnnnnnnnnxxxxxxxxxx,∴由数学归纳法可知:01nnxx,即),2,1(1nxxnn,故nx为单调减少数列。·有界性:只需证明有下界。显然,0nx。或者由数学归纳法∵,3101x34612xx,310623xx,396106634xx,33661nnxx,∴nx有下界。于是,由单调有界收敛准则知:存在极限nnxlim。(2)求极限:设axnnlim,则由16nnxx求极限可得aa6,即0)3)(2(62aaaa,解得:3,2a。注意到0nx,故3a。■―――――――――――――――――――――――――――――――――――――――――――――习题24连续函数及其性质1.求函数11()1xxfxe的间断点,并说明其类型.【解】显然,当1,0x时,函数无定义,故1,0x均为间断点。∵011)1(lim0**1lim*100eeexxxxxx,∴)(lim0xfx,即0x为第二类间断点,且为无穷间断点。∵eeexxxxxx11)1(lim1lim111,10111)1(lim1lim111eeexxxxxx,∴1)(lim,0)(lim11xfxfxx,即1x为第一类间断点,且为跳跃间断点。■注:*极限四则运算法则,**xe的连续性。2.设221()lim1nnnxfxxx,试求函数()fx的表达式,若有间断点,并说明其类型.【解】∵,1||,,1||,1,1||,0lim2xxxxnn∴,1||,1,1||,0,1||,111lim22xxxxxnnn即。1||,,1||,0,1||,)(xxxxxxf由图形易知:1x为第一类间断点,且为跳跃间断点。■3.设21cos,0,(),0,xxfxxaxx要使()fx在,内连续,确定常数a.【解】显然,函数在),0(),0,(内为初等函数,故连续。只需讨论分界点0x处函数的连续性。∵axaxfxx)(lim)(lim200,01coslim)(lim00xxxfxx(无穷小与有界函数积),∴当0a时,()fx在,内连续。■4.讨论sin,0,()1,0,2(11),0xxxfxxxxx的连续性.【解】显然,只需讨论分界点0x处函数的连续性。∵1sinlim)(lim00xxxfxx,1112lim)11(2lim)(lim000xxxxfxxx,∴)0(1)(lim0fxfx,即()fx在,内连续。■5.求下列极限:0ln(1)(1)limxxx(为常数);【解】方法1由等价无穷小可得:xxLx0lim。方法2由重要极限与连续性可得:exxLxxxxln)1(limln)1ln(lim1010。■sinsin(2)limxaxaxa;【解】由三角函数公式、重要极限与连续性可得:aaxaxaxaxaxaxLaxaxaxcos22sinlim2coslim2sin2cos2lim。■0(3)limxxxeex(,为常数).【解】显然,当时,0L。当时,xexexexeLxxxxxxx1lim1lim)11(lim000xxxxxx00limlim。■6.设函数()fx在0,2上连续,且(0)(2)ff,证明在0,上至少存在一点,使得()()ff.【解】作辅助函数)()()(xfxfxF,则∵()fx在0,2上连续,且(0)(2)ff,∴],0[)(CxF。∵)()0()0(ffF,)0()()2()()(ffffF)0(F,∴①当)()0(ff时,可取,0,满足0)(F;②当)()0(ff时,0)()0(FF,由零点定理可知:存在),0(,使得0)(F,即()()ff


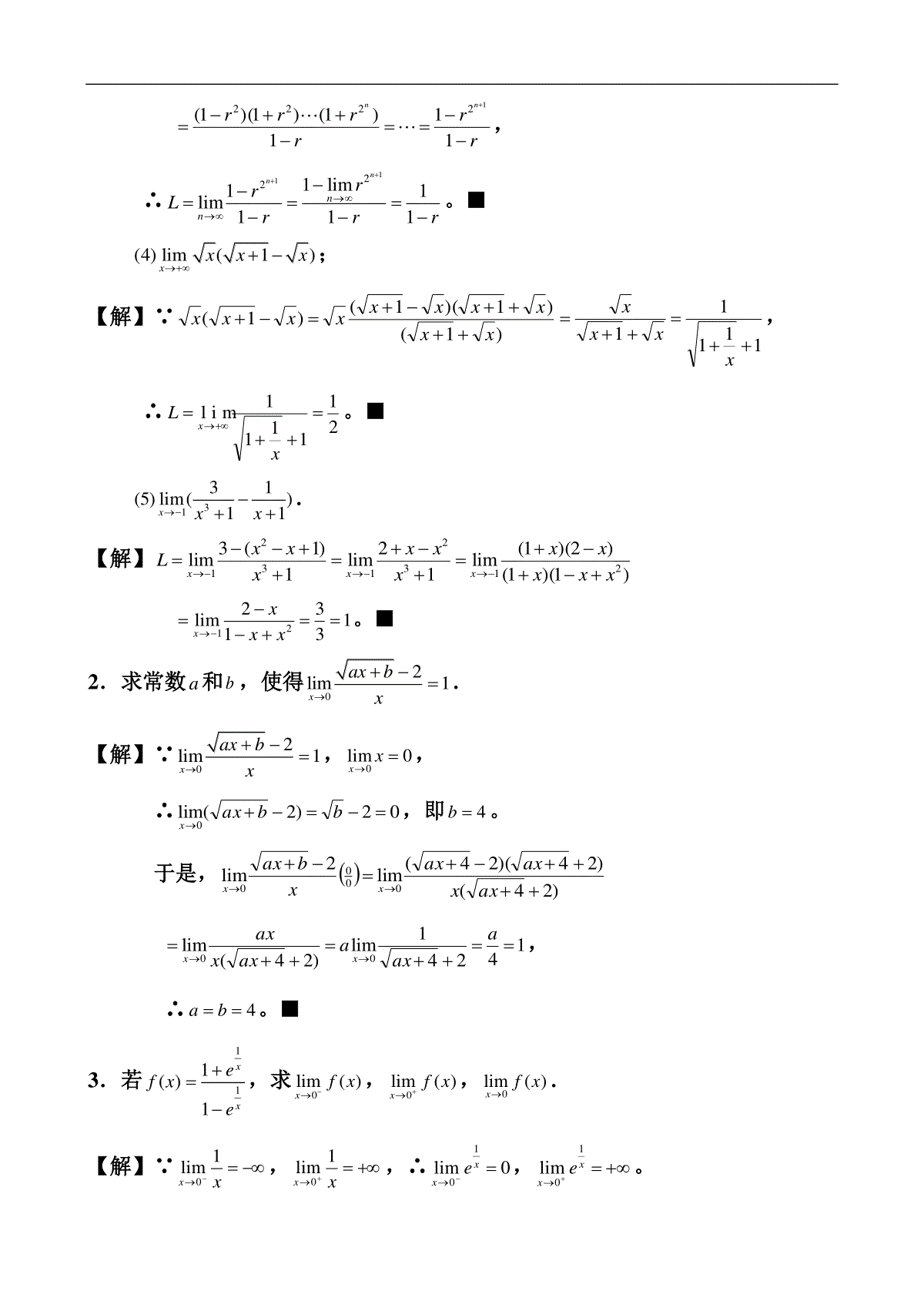

 三七文档所有资源均是用户自行上传分享,仅供网友学习交流,未经上传用户书面授权,请勿作他用。
三七文档所有资源均是用户自行上传分享,仅供网友学习交流,未经上传用户书面授权,请勿作他用。
本文标题:合肥工业大学高数习题册上册答案详解
链接地址:https://www.777doc.com/doc-4534408 .html